These Are the Most Beautiful Equations in Mathematics

To mathematicians, equations are art. Just as many are moved by a painting or piece of music, to those who appreciate and understand math, expressions of numbers, variables, operations and relations between quantities can be just as compelling.

As is the case with artistic beauty, mathematical beauty is in the eye of the beholder. One mathematician may prize simple-to-state, succinct equations, while another may favor the opposite. And just as some favor modern art while others prefer medieval works, both ancient and contemporary equations are admired for their cleverness, power and possibility.

Here are some mathematicians’ selections of the most beautiful math equations, as well as their own words on what factors make them so exquisite.

On supporting science journalism

If you’re enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.

Loewner Differential Equation

Some equations are beautiful because they reveal unexpected relationships between different subjects. The Loewner differential equation, introduced by Charles Loewner in 1923, describes the time evolution of a family of conformal (angle-preserving) functions defined on subsets of the complex plane. The input for the equation is a continuous function W(t), called the driving function.

Nearly 80 years later, in 1999, Oded Schramm discovered that the solution to the Loewner equation has special symmetries when the driving function is taken to be Brownian motion, a random function that is a central object of study in probability theory. Building on Schramm’s discovery, it was proven that the solution to the Loewner equation for this choice of driving function, called Schramm-Loewner evolution, describes the large-scale behavior of various critical models in two-dimensional statistical mechanics. This application of the Loewner equation in probability theory was completely unexpected prior to Schramm’s work. It revolutionized the mathematical study of statistical mechanics and has led to some of the most exciting breakthroughs in mathematics in the past two decades. —Ewain Gwynne, University of Chicago

Gauss-Bonnet Equation

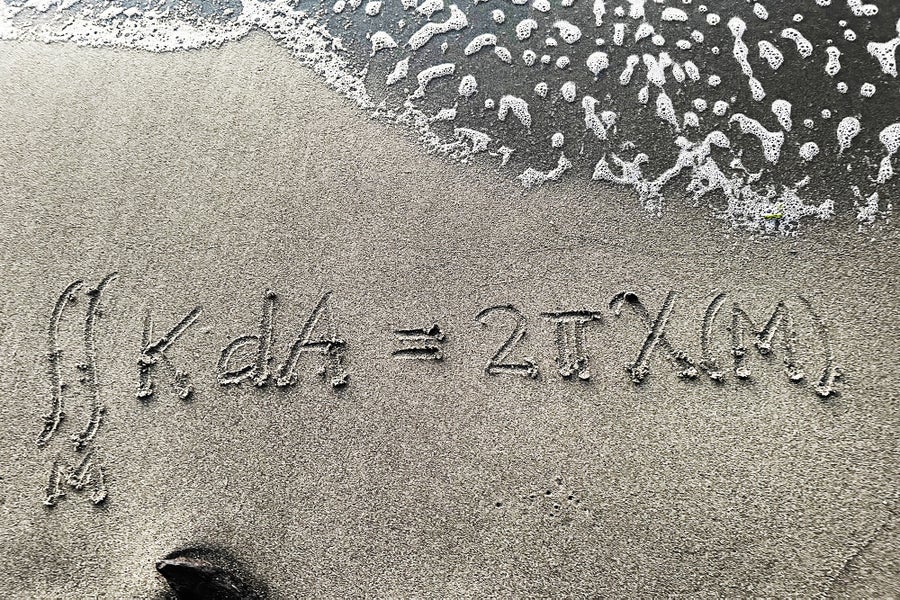
The Gauss-Bonnet formula is a beautiful equation in differential geometry that asserts the equality of the integral of the Gauss curvature over a surface and a constant multiplied by the Euler characteristic of the surface. The equation is remarkable because it relates two very different quantities: the curvature of the surface on the one hand and the topology of the surface on the other hand. The curvature of a surface at a point is a measure of the bending of the surface at that point, or how much the surface deviates from being a plane. The Euler characteristic is a global topological invariant of the surface that describes the topological structure of the surface regardless of how it is bent. The equation is surprising because, for example, it implies that if you continuously deform a surface, the total curvature will remain unchanged. Versions of the Gauss-Bonnet equation were first formulated by Carl Friedrich Gauss and Pierre Ossian Bonnet in the first half of the 19th century, and it remains one of the most beautiful and striking equations in geometry. Some beautiful aspects of this equation are the simplicity of its expression and the profoundness of what it says. —Ailana Fraser, University of British Columbia

Sobolev Inequality

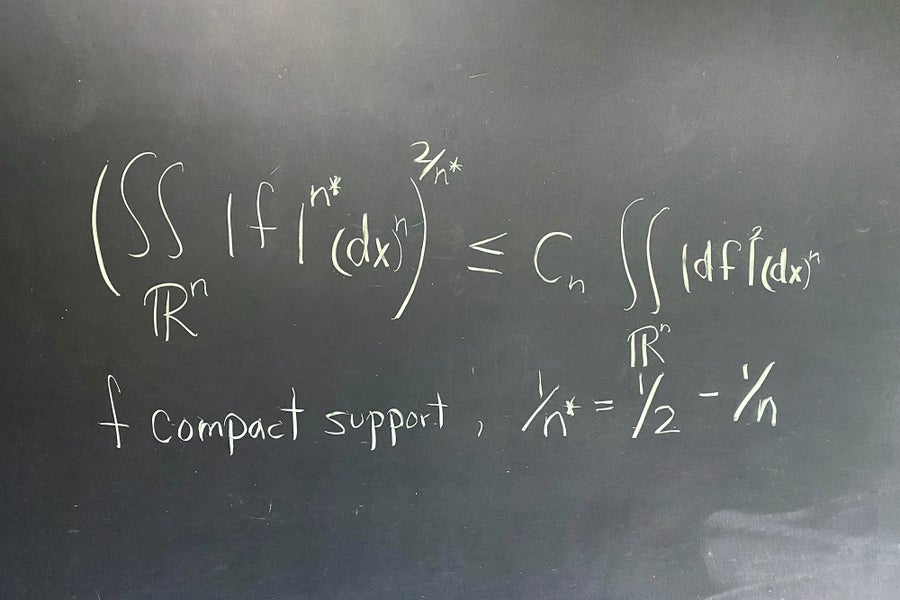
I love inequalities. The Sobolev and related inequalities estimate functions in terms of derivatives and form the basis for our understanding of partial differential equations. The failure of the inequality for n = 2 relates to properties special to n = 2. —Karen Uhlenbeck, University of Texas at Austin

Riemann-Roch Equality

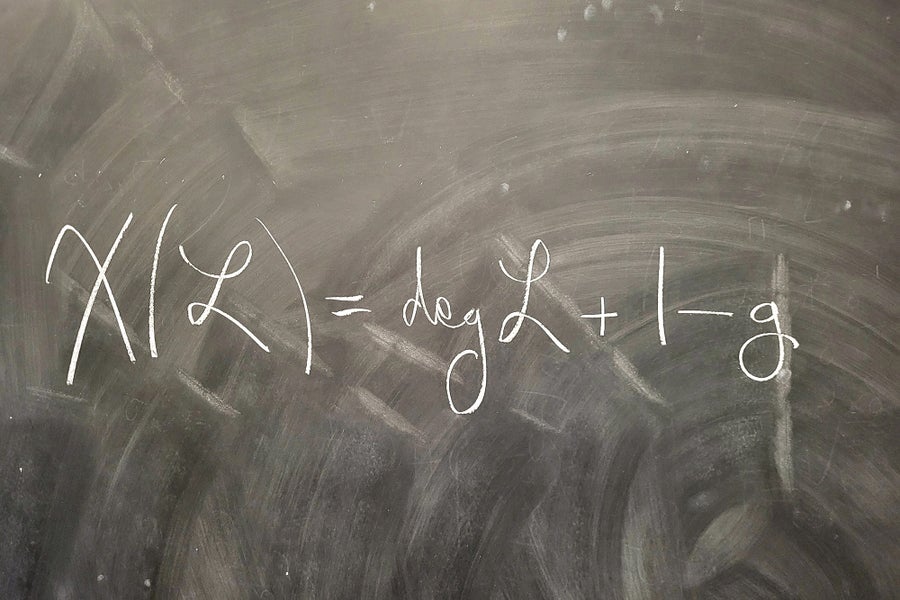
A Riemann surface is a compact orientable surface. Riemann surfaces are distinguished by the number of holes or handles they admit. This number is called the genus, and it is denoted by g. For instance, a Riemann surface of g=0 is a sphere, and a Riemann surface of g=1 is a torus, or doughnut. A complex Riemann surface is a Riemann surface with a complex structure on it. In other words, it is also a complex manifold.

A divisor on a Riemann surface is a choice of finitely many points. The degree of a divisor is the number of points in it. It is denoted by “deg.” A priori, a divisor depends simply on topological information, as does the degree of the divisor. Once a Riemann surface is equipped with a complex structure, however—in other words, when it is considered a complex manifold—then one may ask questions about that complex structure, such as whether there is a meromorphic function whose zeros or poles agree with a given divisor. This leads to a version of the classical Mittag-Leffler problem. One of the simplest invariants that are determined by the complex structure is the holomorphic Euler characteristic of a divisor, which, in a way, is a correction term for the Mittag-Leffler problem on this particular Riemann surface. For a divisor ℒ, its holomorphic Euler characteristic is denoted by χ(ℒ).

Now we are ready to state the Riemann-Roch equality. Consider a complex Riemann surface of genus g and a divisor ℒ on it. The equation is χ(ℒ) = degℒ + 1 – g.

This simple-looking equation has far-reaching consequences. Among other things, it implies that the holomorphic Euler characteristic only depends on topological information.

As for what makes an equation beautiful, I would say that the ones I find intriguing are those that either reveal something unexpected or connect seemingly unrelated invariants. —Sándor Kovács, University of Washington

Isomorphism between Graded Rings

I find this equation beautiful because it’s novel and surprising, and information clearly flows from the right-hand term (RHT) to the left-hand term (LHT). Indeed, the LHT is manifestly geometric, while the RHT is purely algebraic. Furthermore, because the RHT is calculable, this equation gives a classification of manifolds, an enterprise of intense study. Lastly, this result was a first of its kind, whose proof techniques prompted creative new approaches to and perspectives in many areas of math. —David Ayala, Montana State University

An Infinite Sum

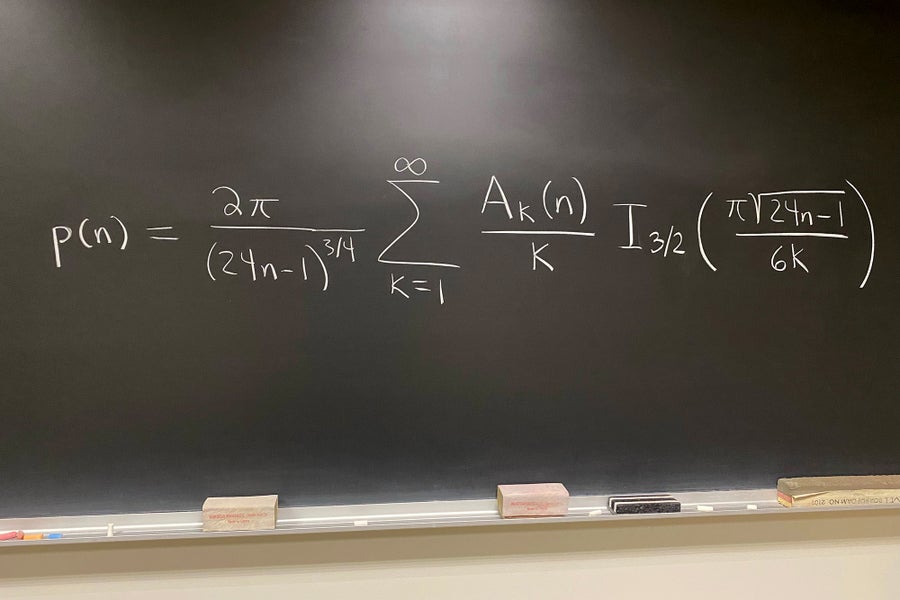
I don’t think there’s a single most beautiful mathematical equation, but this one stands out to me. I showed a photograph of it to a young child while working on this piece. We first talked about what an equation is (a mathematical statement relating two things with an equals sign, such as 4 = 2 + 1 + 1), and they asked why this equation is so big. We talked about how the right-hand side is visually the big side but the left-hand side isn’t—how the right-hand side is a sum (like 2 + 1 + 1) but of an infinite number of terms and how the left-hand side has to do with integer partitions in number theory. For example, there are five partitions of n = 4 (4, 3 + 1, 2 + 2, 2 + 1 + 1, 1 + 1 + 1 + 1), so the partition function p(n) evaluated at n = 4 is 5 (p(4) = 5).

This important and seemingly basic function having to do with adding and counting is beautifully and perhaps unexpectedly complex. For example, p(100) is more than 190 million, but surely the way to figure that out isn’t by writing out the millions of partitions of n = 100 and counting how many there are. The right-hand side of this equation is an exact formula for p(n) thanks to the 1937 work of Hans Rademacher, who extended related earlier work of G. H. Hardy and Srinivasa Ramanujan. Some may view the (big) right-hand side, an infinite sum involving sums of complex (imaginary) numbers (A_k(n)), fractional powers (³/₄ and the square root), the transcendental number pi, and more, as the opposite of beautiful or as intimidating—especially given that it replaces the (visually small) left-hand side that even a child can understand. It’s the beauty of analytic number theory and Rademacher and Hardy and Ramanujan’s work that shows that this formula for p(n) exists—a feat in and of itself—and that it turns out to be practical for computing p(n) by truncating the infinite sum and essentially rounding. It’s beautiful that the infinite sum that appears here converges, meaning it sums to something finite, a real number that counts something of importance, and doesn’t keep on accumulating—not to mention the mathematical legacy, further research and connections to other areas that persist today, now close to a century later. —Amanda Folsom, Amherst College

The Euler Product Formula

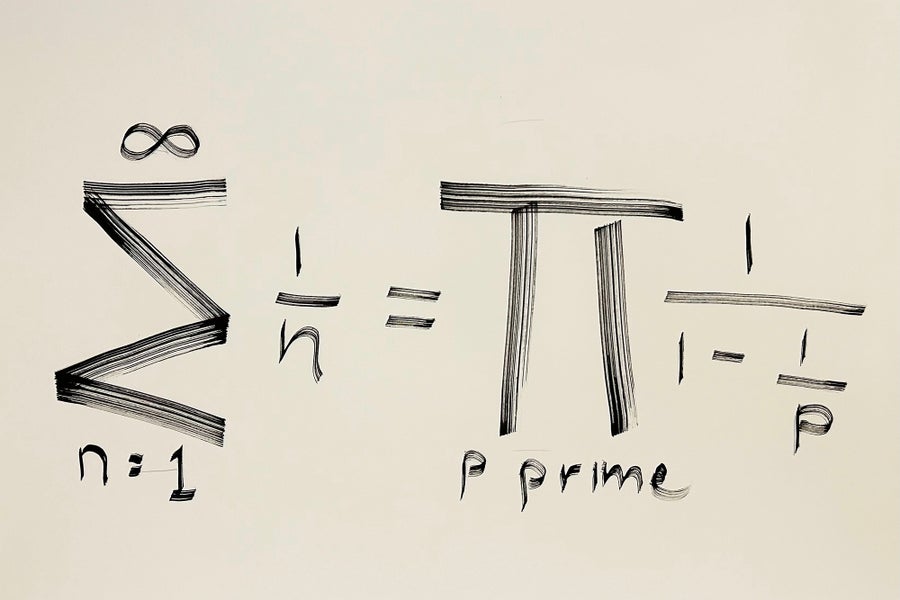
It is hard to pick a single most beautiful equation in mathematics, but I think that the Euler product formula would be near the top of any such list. Let me extol the virtues of this formula. First, it is simple. It only involves the operations of addition, subtraction, multiplication and division. Second, it is fundamental: it expresses a deep relationship between counting numbers on the left-hand side and prime numbers on the right-hand side. Third, it is surprising and unexpected, intertwining the basic operations in a very novel way. Fourth, it is useful and powerful. Just as a start, it gives another proof of the infinitude of primes. The left-hand side is a divergent series. If there were only finitely many primes, the right-hand side would be finite, a contradiction. One can also squeeze deeper information about primes from the equation. For instance, one can use the Euler product formula to prove that the sum of the reciprocals of the primes diverges. This is a result that goes way beyond Euclid’s classic proof of the infinitude of primes. Fifth, the formula is thoroughly current. It still plays a major role in number theory and is one of the key tools in understanding the distribution of prime numbers, even to this day. Sixth, it is accessible: it has an elegant proof that uses the fact that integers can be uniquely factored, along with neat properties of geometric series. —Richard Schwartz, Brown University

A Reifenberg FlatSet

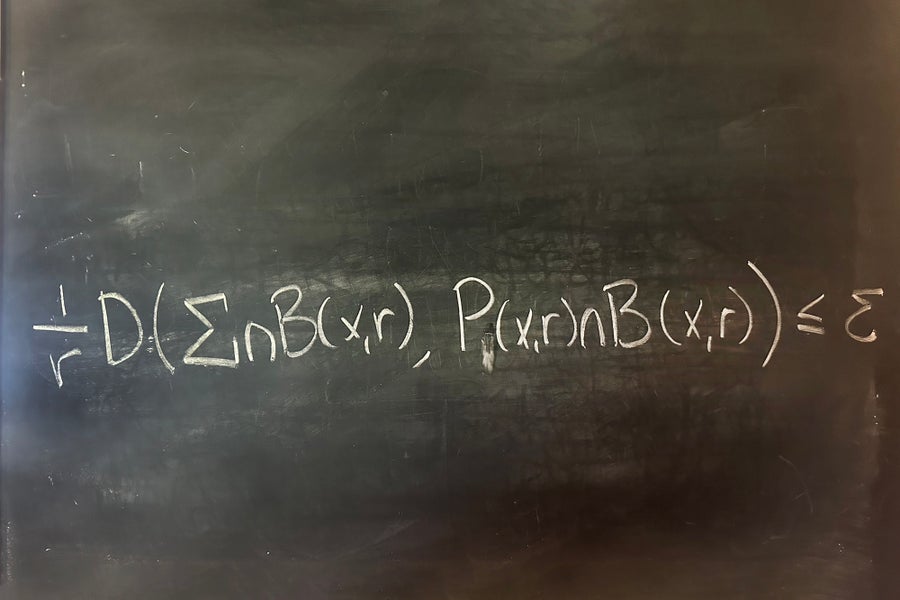
A set Σ whose points satisfy the inequalities in the picture (they are hidden, but there are two!) is called Reifenberg flat. As usual, the symbol ε in mathematics represents a small quantity; we are essentially saying that in every ball B(x,r), at every scale and location, there exists a plane P(x,r), whose distance from our set is very tiny (εr).

Peter Reifenberg proved in 1960 that topologically, these sets are disks. (That’s a good thing: no rips, holes or weird twists.) In fact, as more recent results show, asking these sets to be a little extra flat leads to smoother parameterizations. Being flat is quite demanding for a set, but it sure pays off in regularity. The interplay between flatness (geometry) and regularity (analysis) is at the heart of everything I love about math, and it is something that most readers already know but may not realize: we learn early on that derivatives give us tangents!

I don’t think that the beauty in mathematics lies in the equations, although I am certain that good handwriting can make miracles. I overwhelmingly find it in the ideas that are capable of bringing two mathematical universes together to create room for new concepts while enhancing our understanding of old ones. —Silvia Ghinassi, University of Washington

(¹/_p) + (¹/_q) + (¹/_r) = 1

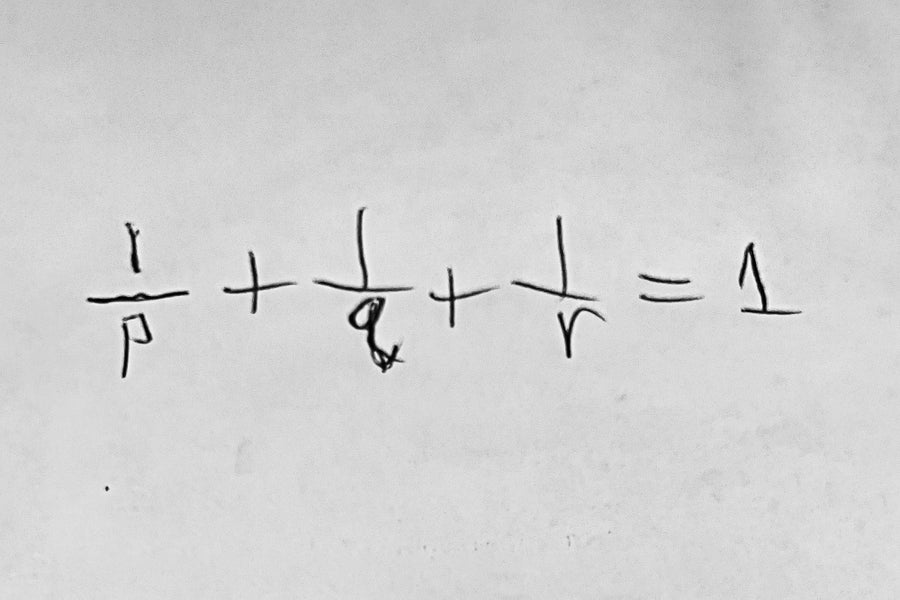
My favorite equation is (¹/_p) + (¹/_q) + (¹/_r) = 1. Its only solutions in positive integers are p = q = r = 3; p = 2, q = r = 4; and p = 2, q = 3, r = 6. This equation pertains to a beautiful class of groups called Coxeter, or reflection, groups and marks the crucial boundary between finite and infinite groups of this kind. Its solutions are intimately related to the only ways of tiling the plane by regular polygons, namely by equilateral triangles, squares or hexagons. —Monty McGovern, University of Washington